Magnetic moment

A magnetic moment, also known as magnetic dipole moment or magnetic torque, is a unit that describes the strength of a dipole. It shows the relationship between the strength of the field and the highest achievable mechanical torque. A distinction is made between a magnetic spin moment, which specifies the force created by the self-rotation of the electrons, and a magnetic orbital moment. The latter in turn is used to characterize the movements of electrons around their atomic nucleus. Both dipole moments are linked by interactions - the so-called spin-orbit interactions.

How does the magnetic torque work in a magnetic field?

The torque of the magnetic field of an external magnet act on a magnetic moment and rotates it in its field direction. The resulting angle between the magnetic torque and the direction of the field influences the prevailing energy. The cause of the creation of a magnetic moment can be, on the one hand, electrical circulating currents and, on the other hand, the intrinsic angular momentum of the spins.

For example, if one imagines a closed current path, the magnetic moment would be in the center of the circular area that is created and depends on the direction of the current. Something similar can be observed at the atomic level, since the atomic nuclei are orbited by electrons - like how the planets of our solar system in the universe orbit the sun. The intrinsic rotation of the electrons is called spin.
The movement around the atomic nucleus creates a magnetic dipole moment. The sum of all these moments in a magnetic body would in turn represent the total magnetic force - expressed in the unit of the magnetic moment.

It is also possible that the magnetic moments of different atoms are linked to one another. This happens, for example, in the case of magnetization of a ferromagnetic body. If such a magnet is located close to a sufficiently strong, external magnet or if an electrical voltage has been applied to it, various processes are triggered on the atomic spectrum level.

The elementary magnets in the ferromagnetic material (the smallest magnetic structures that resemble tiny bar magnets and correspondingly have their own magnetic fields) are initially arranged irregularly. The body is not yet magnetic, as the individual smallest magnetic fields of the elementary magnets balance and thus neutralize each other. The magnets are in small areas separated by walls, the so-called Weiss districts. If a voltage or an external magnet is applied, the elementary or molecular magnets align themselves parallel to one another and the individual areas merge into one another. This very alignment is based on a force also known as the exchange interaction. It can be explained on the level of quantum mechanics and with the help of the magnetic moment.

Electrically charged electrons with an inherent orbital angular momentum are influenced by electric fields. They therefore arrange themselves in such a way that their energy is as low as possible. If the orbital angular momentum is now reduced, the above-mentioned spin-orbit interaction is also reduced. The spins of the electrons influence the magnetic moment, and the molecular magnets turn parallel to each other towards the external electric or magnetic field. A new magnet was created in this way, whose field lines converge with the already existing magnetic field and strengthen it.

In some materials it is possible to shut down the spin-orbit interaction particularly quickly - depending on the respective crystal structure of the substance. If this is the case, one speaks of a soft magnetic material that can be magnetized as quickly as it can be demagnetized. Examples for this are:

  • Iron
  • Cobalt
  • Nickel
  • Iron-cobalt-nickel based alloys
  • Soft ferrites (nickel-zinc or manganese-zinc compounds)
  • Powder materials

Hard magnetic material is used when the magnetism is more difficult to achieve due to a higher directional dependence, but also lasts when the external permanent magnet or the electric field has been removed. Hard magnetic are among others:

  • Neodymium-iron-boron magnets
  • Martensitic steels
  • Hard ferrites based on strontium or barium
  • Iron-copper-chromium alloys

How can the magnetic field strength of an elementary magnet be determined using the magnetic moment?

To calculate the magnetic moment, two factors have to be related to each other in a flat conductor loop: the circulating current itself and the area it surrounds. The formula for calculating the magnetic moment is as follows: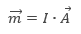

If you consider the magnetic moment in a longer coil, the number of turns n must also be included in the calculation:

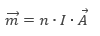If the magnetic moment of an individual particle is to be calculated, the applied charge must be related to the respective angular momentum of the particle.
The following formula is to be used for this:

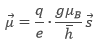The μ denotes the magnetic moment of the particle, q the applied charge and μB the so-called Bohr moment. This is the moment that corresponds to the orbital angular momentum of an electron on Bohr's first orbit of the hydrogen atom. The s indicates the prevailing spin.
The fixed units e (elementary charge), g (Landé factor, which describes the relationship between angular momentum and magnetic moment) and h (Planck's quantum of action, which fixes angular momentum) must also be included in the calculation.


back to overview