Ferri- and antiferromagnetism
Ferrimagnetism and antiferromagnetism are two magnetic properties of materials. In contrast to antiferromagnetic materials, ferrimagnetic materials are strongly attracted to a magnetic field. Paramagnetism, ferromagnetism and diamagnetism are other magnetic properties of matter.
In particular, the latter three properties are well known, but not all materials can be fully characterized. So there are substances that have to be divided into antiferromagnetism and ferrimagnetism. The two properties can be described by superimposing two ferromagnetic sublattices polarized against each other. For example, manganese oxide has two adjacent electron spins. These elementary magnets are aligned antiparallel, forming two levels of spins, which are in turn parallel to each other. This is a typical example of antiferromagnetism. In such a material, the two ferromagnetic sublattices and their magnetic properties cancel each other out.
In ferrimagnetism one of these sublattices or its magnetic property is stronger than that of the other sublattice. Furthermore, it is not mandatory that the different sublattices follow an anti-parallel alignment. To fully understand the meaning and mode of action of antiferromagnetism and ferrimagnetism, one is best acquainted with the foundations of ferromagnetism, the most well-known magnetic property:
FerromagnetismThis alignment is stabilized by the exchange interaction of the electron spins aligned in parallel in a ferromagnetic material. As a result, a ferromagnetic material can be magnetized. When the ferromagnet is fully magnetized, all the electron spins in the matter are aligned in parallel. A higher degree of magnetization can not be achieved.
In an antiferromagnetic substance, however, there is also such a maximum degree of magnetization, but in the sublattice in each case. This means that the atomic spins only partially align with the external magnetic field - all other spins align in exactly the opposite orientation. This can be compared with the so-called Weiß's districts, which arise in an incomplete magnetization and in particular in the demagnetization of a ferromagnetic material. Although the electron spins within a Weiss district are aligned in parallel, there is no parallel alignment between the various districts. In an antiferromagnetic material, the districts overlap to form the sublattices just mentioned. The antiferromagnet compensates for the magnetic moments of all sublattices. Ferrimagnetism in turn outweighs the entire magnetic moment of a given orientation or sublattice.
This results in the following effect: An antiferromagnet does not amplify a magnetic field, a ferrimagnet in turn can amplify a magnetic field - it behaves basically like a weak ferromagnet.
For ferromagnetic materials there is a substance-specific Curie temperature, which indicates from which temperature a ferromagnetic material becomes a paramagnetic material. The reason for this is that when the Curie temperature is exceeded, the orientation of the spins is destroyed by the thermal motion of the individual atoms. For the magnetic susceptibility X of a substance, the Curie constant C applies:
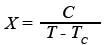 Something similar also exists for antiferromagnets, but not with the Curie temperature or the Curie constant, but with the so-called Neel temperature or the Neel constant N:
Something similar also exists for antiferromagnets, but not with the Curie temperature or the Curie constant, but with the so-called Neel temperature or the Neel constant N:
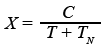
 Thousands of products in stock
Thousands of products in stock